Henderson Hasselbalch Equation – Principle, Uses, Practice, Examples

The Henderson-Hasselbalch equation is a handy formula used in chemistry and biology to estimate the pH of a solution, especially when dealing with buffers—mixtures that resist changes in acidity or alkalinity. Think of it like a cheat sheet for predicting how acidic or basic a solution will be based on the balance between a weak acid and its conjugate base (or a weak base and its conjugate acid). The equation itself is pretty straightforward: pH = pKa + log([base]/[acid]). Here, pKa is a measure of the acid’s strength, while [base] and [acid] represent the concentrations of the base and acid forms in the solution.

This equation is super useful in real-world scenarios. For example, in your bloodstream, buffers like bicarbonate rely on this balance to keep your pH stable, preventing drastic shifts that could harm cells. It’s also a go-to tool in labs for designing experiments or in medicine when figuring out how drugs get absorbed. The catch? It works best under “ideal” conditions, assuming things like temperature or ion strength don’t throw off the measurements. Even with its limits, though, the Henderson-Hasselbalch equation remains a cornerstone for understanding how acids and bases behave together—no lab coat required.

What is the henderson hasselbalch equation​?

A mathematical formula connecting the pH of a buffer solution to the concentration of its acidic and basic components is the Henderson–Hasselbalch equation.

It is expressed as:

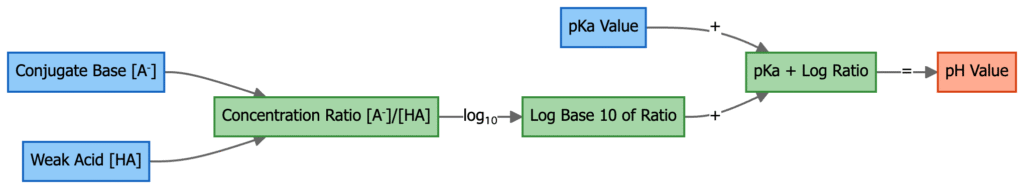
pH = pKₐ + log([A⁻]/[HA])

where pKₐ is the acid dissociation constant, [A⁻] is the concentration of the conjugate base, and [HA] is the concentration of the acid.

In chemistry and biology, this equation is extensively applied to predict the pH of buffer solutions when known amounts of the acid and its conjugate base are known.

It helps especially when creating buffer solutions with a specified pH by changing the acid to base ratio.

The equation makes the assumption that the acid and its conjugate base are in a dilute solution and that under the conditions of application the acid dissociation constant (pKₐ) is known and constant.

Swedish physiologist Karl Albert Hasselbalch in 1909 and American biological scientist Lawrence Joseph Henderson in 1908 separately devised the Henderson–Hasselbalch equation. Henderson developed an equation to determine the hydrogen ion concentration of a bicarbonate buffer solution; subsequent re-expression of this equation by Hasselbalch in logarithmic terms produced the equation we employ now.

The equation bears the names of both scientists to respect their contributions to knowledge of acid-base equilibrium in biological systems.

Practically, the equation is used in environmental research, biochemistry, and medicine to investigate and control pH levels in biological fluids, soil, and water systems.

Although the equation offers a good estimate, variables such ionic strength, temperature, and the presence of other compounds that could impact pH might reduce its accuracy.

Notwithstanding its constraints, the Henderson–Hasselbalch equation is still a basic instrument for pH comprehension and control in chemical and biological systems.

Definition of Henderson Hasselbalch Equation

The Henderson–Hasselbalch equation is an approximate relationship that calculates the pH of a solution containing a weak acid and its conjugate base from the acid’s pKa and the log of their concentration ratio

Objectives of Henderson Hasselbalch Equation

• A basic instrument in acid-base chemistry, the Henderson-Hasselbalch equation helps one to calculate and grasp pH in many solutions.
• It enables the determination of pH, pOH, [H₃O⁺]ₜₒₜ, [OH⁻]ₜₒₜ, [H₃O⁺]ₓₓₓ, and [OH⁻]ₓₓₓ in solutions containing strong acids or bases, given their initial concentrations.
• The equation explains how buffer solutions—whether acidic or basic—resist major pH changes with the addition of modest quantities of acid or base, hence preserving homeostasis in biological systems.
• It offers a way to change the ratio of acid to conjugate base concentrations such that buffer solutions with chosen pH values are created.
• The equation helps one to grasp the idea of buffer capacity—that is, the capability of a buffer solution to withstand pH fluctuations—as well as how this capacity fluctuates with the concentrations of the acid and base components.
• By examining the acid dissociation constant (Ka) and base dissociation constant (Kb) of the conjugate acid-base pairs, it helps one forecast the acidic, basic, or neutral character of aqueous salt solutions.
• By means of their respective Ka and Kb values, the equation enables the assessment of the relative strengths of conjugate acids and bases, therefore offering understanding of their protonation and deprotonation tendencies.
• Understanding enzyme activity and molecular interactions depends on knowing the protonation state of biomolecule functional groups, hence it is rather important under physiological pH settings especially.
• Widely used in many disciplines, including biochemistry, pharmacology, and environmental research, the Henderson–Hasselbalch equation studies and controls pH levels in biological fluids, pharmacological formulations, and ecological systems.

Factors Affecting Henderson Hasselbalch Equation

Estimating the pH of buffer solutions is a common usage for the Henderson-Hasselbalch equation. Still, various elements can affect its accuracy:

• Ionic Strength
  • The equation assumes perfect behaviour in which case activity coefficients are unity.
  • Real solutions, particularly in high ionic strengths, interactions between ions can change activity coefficients, therefore influencing the pH computation.
  • In concentrated solutions, deviations from optimal behaviour become important and cause pH estimates to be off.
• Temperature
  • Acids and bases have temperature-dependent pKa values.
  • Usually declining pKa as temperature rises, this might change the pH of a buffer solution.
  • These temperature-induced variations are not taken into consideration in the Henderson–Hasselbalch equation, so pH estimate may suffer from mistakes.
• Concentration of Buffer Components
  • When the concentrations of the acid and its conjugate base are rather greater than the concentrations of H⁺ and OH⁻ ions, the equation is most correct.
  • The presumption that the self-ionization of water may be disregarded becomes false at extremely low concentrations, therefore influencing the pH computation.
• Buffer Capacity
  • Buffer capacity—the capability of a buffer to resist pH changes upon addition of an acid or base—is not immediately accounted for by the equation.
  • Although similar, buffer capacity is determined by absolute acid and base concentrations rather than only their ratio.
• Self-Ionization of Water
  • H₂O dissociation becomes quite important at pH levels close to 7.
  • The equation implies this contribution is small, which might not be accurate in dilute solutions.
• Polyprotic Acids
  • The equation only applies for acids that may give more than one proton (e.g., H₂CO₃) if the pKa values for subsequent dissociations vary by at least 3 units.
  • Should this requirement not be satisfied, the equation might not fairly estimate pH.
• Conjugate Base Strength
  • The equation treats the conjugate base as sufficiently strong to take protons.
  • The usefulness of the equation may be limited in circumstances when the conjugate base is weak as the buffer may not sufficiently resist pH variations.
• Salt Dissociation
  • The equation makes full dissociation of salts in solution as assumption.
  • Variations from predicted pH levels might result from incomplete dissociation.
• pH Range of Buffer
  • When the pH falls inside ±1 unit of the pKa, the equation is most consistent.
  • Beyond this range, the buffer’s resistance to pH decreases and the predictions of the equation get less accurate.
• Assumption of Ideal Behavior
  • The equation considers perfect behaviour in which every species exists in their natural conditions.
  • Deviations from ideal behaviour can happen in actual solutions and cause pH estimations to be inaccurate.
• Environmental Factors
  • pH may be changed by elements like pressure and the presence of other solutes.
  • The equation’s accuracy may suffer depending on these factors not taken into consideration.
• Limitations in Biological Systems
  • In biological systems, pH may be changed by elements like ionic strength and protein content.
  • The equation could not be able to adequately represent these complexity, which would cause possible pH estimate errors.
• pKa Determination
  • Reliability of the equation depends on exact pKa value determination.
  • Variations in experimental circumstances might cause differences in pKa values, therefore influencing pH estimates.
• Buffer Preparation
  • The equation lets components be pure and presupposes proper preparation of buffers.
  • Variations from planned pH values in preparation might result from mistakes or contaminants.
• Use in Complex Mixtures
  • Interactions among components in complicated mixes including several acids and bases may cause the equation to fail in precisely determining pH.
  • Under such circumstances, more complex models might be needed.
• Non-Ideal Solutions
  • The equation takes ideal behaviour of solutions as assumption.
  • In non-ideal solutions—that is, ones with strong concentrations or interactions between solutes—the equation’s predictions may be less exact.
• Dynamic Changes
  • The equation offers a constant pH calculation.
  • The equation might not fairly represent pH changes in dynamic systems where pH fluctuates over time, including those of metabolic activities.
• Buffering Capacity at Extremes
  • A solution’s buffering capacity falls at very high or low pH levels.
  • The equation might not fairly forecast pH under these severe circumstances.
• Concentration Range
  • When the concentrations of the acid and base fall within a specific range, the equation is most exact.
  • Outside this range, the predictions of the equation could lose dependability.
• Analytical Techniques
  • The calculation takes precise and dependable pH measurements as assumption.
  • pH measurements may vary depending on analytical method used.
• Environmental Conditions
  • pH may be influenced by things including pollution and humidity.
  • These environmental factors—which might affect pH readings—are not included into the calculation.
• Interference from Other Species
  • Other species present in a solution can affect pH readings.
  • The equation makes the assumption that just the components of acid and base will be present, which might not be the case in complicated combinations.
• Long-Term Stability
  • Components of the buffer solution could change pH by degrading or reacting over time.
  • According to the equation, the buffer’s constituent parts

Henderson Hasselbalch Equation Derivation/Principle/Theory

The Henderson–Hasselbalch equation provides a quantitative relationship between the pH of a buffer solution, the acid dissociation constant (pKa) of the acid, and the ratio of the concentrations of the conjugate base ([A⁻]) to the weak acid ([HA]).

It is derived from the acid dissociation equilibrium of a weak acid (HA) in water:

• HA ⇌ H⁺ + A⁻
• The acid dissociation constant (Ka) is expressed as:
  • Ka = [H⁺][A⁻] / [HA]

Taking the negative logarithm of both sides:

• -log(Ka) = -log([H⁺][A⁻] / [HA])
• This simplifies to:
  • pKa = pH – log([HA]/[A⁻])

Rearranging the equation:

• pH = pKa + log([A⁻]/[HA])

This equation is valid under certain assumptions:

• The acid is weak and does not dissociate completely.
• The solution is dilute, and the self-ionization of water is negligible.
• The salt of the conjugate base is fully dissociated in solution.

The Henderson–Hasselbalch equation is widely used to:

• Calculate the pH of buffer solutions.
• Determine the pKa of weak acids and bases.
• Prepare buffer solutions with desired pH values.
• Analyze the buffering capacity of solutions.

It is important to note that the equation assumes ideal behavior and may not be accurate for very dilute solutions or in the presence of strong acids or bases.

Henderson Hasselbalch Equation

For a weak acid:
pH = pKa + log([conjugate base]/[weak acid])

For a weak base:
pOH = pKb + log([conjugate acid]/[weak base])

where,

• pK_a= dissociation constant of acid
• pK_b= dissociation constant of base

Applications of Henderson Hasselbalch Equation

• Preparation of Buffer Solutions
  • By changing the acid to conjugate base concentration ratio, the equation helps to construct buffer solutions with certain pH values.
  • In laboratory environments when exact pH control is needed for tests, this is absolutely vital.
• Physiological and Biochemical Uses
  • Enzyme activity and metabolic events in biological systems depend on a constant pH.
  • The formula clarifies and preserves the pH of biological fluids such as blood and intracellular surroundings.
• Pharmaceutical Formulation
  • Pharmaceutical drugs are developed using this equation, which guarantees their pH will remain stable and effective.
  • For medications given topically or intravenously especially, this is rather crucial.
• Environmental Chemistry
  • Monitoring pollution and aquatic life depends on knowing the pH of natural water bodies, hence it helps in their evaluation.
  • The equation clarifies how buffering capacity of natural waterways resists acid rain.
• Titration Curve Analysis
  • Titration curves of weak acids and bases are interpreted using the equation, therefore revealing their dissociation properties.
  • In analytical chemistry, this is useful for spotting unidentified compounds.
• Chemical Nature of Protein
  • It aids in the determination of amino acid residue ionisation states in proteins, therefore affecting protein structure and operation.
  • In the research of enzyme processes and protein interactions, this is crucial.
• Clinical Investigations
  • Blood gas readings are interpreted using the equation, which helps diagnose respiratory and metabolic diseases.
  • It aids in patient assessment of their acid-base balance.
• Agricultural Chemicals
  • It helps one to grasp soil pH and how it affects plant nutrition availability.
  • The equation guides soil additions to maximise crop output.
• Food chemistry
  • Food product pH is controlled using the equation, therefore influencing flavour, preservation, and safety.
  • It helps create things like dairy products, jams, and sauces.
• Educational Tool
  • In teaching acid-base equilibria and buffer systems, the equation functions as an instructional aid.
  • It offers a useful approach to theoretical ideas in biology and chemistry.

Advantages of Henderson Hasselbalch Equation

• Offers a straightforward formula for determining buffer pH without the need to solve intricate equilibrium expressions.
• By approximating mild acid/base ionization as negligible, the necessity for ICE tables is eliminated.
• Facilitates the straightforward calculation of the necessary acid-to-base ratio to create a buffer with the desired pH.
• Establishes a linear logarithmic relationship between pH and pKa, which elucidates the impact of concentration variations on pH.
• Enables the estimation of unknown pKa values when pH and conjugate ratio are measured.
• Is a fundamental instrument in the field of physiology that is used to simulate the buffering systems of blood and cells.
• Aids enzyme and protein investigations by facilitating rapid pH approximation in biological and biochemical assays
• Supports the prediction of ionized versus unionized drug fractions, which is crucial for drug formulation and pharmacokinetics.
• Enhances versatility by applying equally to acidic and basic buffers with a straightforward algebraic sign change
• It is widely used in education and research due to its clarity, which makes acid-base concepts more accessible to students.

Limitations of Henderson Hasselbalch Equation

• Doesn’t take into account activity factors and ionic strength effects, which can lead to wrong pH readings in liquids with lots of ions or strong ions
• It assumes that concentrations and activities are similar, so it needs ideal solution behavior. When solution behavior isn’t ideal, growing mistakes happen.
• It’s only close to the pKa number (usually within one pH unit); estimation mistakes get very big outside of this range.
• Doesn’t take into account the effects of water ionization and hydrolysis, which can lead to mistakes when pH is close to normal or buffers are very weak.
• Assumes that the temperature stays the same and doesn’t take into account that pKa and pH change with temperature.
• It’s not good for strong acids or bases that fully separate because it was made for weak acid/base pairs.
• Not accurate at very low buffer concentrations because dilution changes activity factors and component ratios in a big way.
• Cannot be used directly on polyprotic acids without being changed and each breakdown step having to be looked at separately.
• It doesn’t look at buffer capacity limits, so it can only guess pH and not how resistant the solution is to acids or bases being added.
• Based on a logarithmic approximation, it may be too low or too high for the hydrogen ion concentration compared to accurate estimates, especially when the component ratios are very high or very low.

Examples of Henderson Hasselbalch Equation

• Buffer pH Calculation
  • A solution contains 0.36 M sodium acetate (CH₃COONa) and 0.45 M acetic acid (CH₃COOH). The pKa of acetic acid is 4.8.
  • Using the Henderson-Hasselbalch equation:
    pH = pKa + log([A⁻]/[HA])
    pH = 4.8 + log(0.36/0.45)
    pH ≈ 4.8 + log(0.8)
    pH ≈ 4.8 – 0.0969
    pH ≈ 4.7031
  • Therefore, the pH of the buffer solution is approximately 4.7.
• Buffer pH Calculation with Ammonia and Ammonium Chloride
  • A solution contains 0.7 M ammonia (NH₃) and 0.9 M ammonium chloride (NH₄Cl). The pKa of ammonia is 9.248.
  • Using the Henderson-Hasselbalch equation:
    pH = pKa + log([A⁻]/[HA])
    pH = 9.248 + log(0.9/0.7)
    pH ≈ 9.248 + log(1.2857)
    pH ≈ 9.248 + 0.108
    pH ≈ 9.356
  • Therefore, the pH of the buffer solution is approximately 9.36.
• Buffer pH Calculation with Acetic Acid and Sodium Acetate
  • A solution contains 0.20 M acetic acid (CH₃COOH) and 0.50 M sodium acetate (CH₃COONa). The pKa of acetic acid is 4.76.
  • Using the Henderson-Hasselbalch equation:
    pH = pKa + log([A⁻]/[HA])
    pH = 4.76 + log(0.50/0.20)
    pH ≈ 4.76 + log(2.5)
    pH ≈ 4.76 + 0.3979
    pH ≈ 5.1579
  • Therefore, the pH of the buffer solution is approximately 5.16.
• Buffer pH Calculation with Hydrofluoric Acid and Sodium Fluoride
  • A solution contains 3.0 M hydrofluoric acid (HF) and 2.5 M sodium fluoride (NaF). The Ka for HF is 6.76 × 10⁻⁴.
  • First, calculate the pKa:
    pKa = –log(6.76 × 10⁻⁴)
    pKa ≈ 3.171
  • Using the Henderson-Hasselbalch equation:
    pH = pKa + log([A⁻]/[HA])
    pH = 3.171 + log(2.5/3.0)
    pH ≈ 3.171 + log(0.8333)
    pH ≈ 3.171 – 0.0792
    pH ≈ 3.0918
  • Therefore, the pH of the buffer solution is approximately 3.09.
• Buffer pH Calculation with Benzoic Acid and Sodium Benzoate
  • A solution contains 0.00750 mol benzoic acid (C₆H₅COOH) and 0.00250 mol sodium benzoate (C₆H₅COONa) in 0.500 L. The pKa of benzoic acid is 4.190.
  • Calculate the concentrations:
    [HA] = 0.00750 mol / 0.500 L = 0.015 M
    [A⁻] = 0.00250 mol / 0.500 L = 0.005 M
  • Using the Henderson-Hasselbalch equation:
    pH = pKa + log([A⁻]/[HA])
    pH = 4.190 + log(0.005/0.015)
    pH ≈ 4.190 + log(0.3333)
    pH ≈ 4.190 – 0.4771
    pH ≈ 3.7129
  • Therefore, the pH of the buffer solution is approximately 3.71.

These examples demonstrate how to apply the Henderson-Hasselbalch equation to calculate the pH of buffer solutions composed of weak acids and their conjugate bases.

Henderson Hasselbalch equation practice problems

Practice Problems

Problem 1: pH from concentrations

A solution contains 0.100 M CH₃COO⁻ and 0.400 M CH₃COOH. Calculate the pH. (pKa = 4.76)

Problem 2: Percent undissociated acid

A weak acid has pKa = 7.07. If the solution pH is 7.38, what percentage of the acid remains undissociated?

Problem 3: pH change on adding strong acid

You have 0.500 L of an acetic-acid buffer (total [acid+base] = 0.800 M, pH = 5.000, pKa = 4.752). You add 0.100 mol HCl (no volume change). What is the new pH?

Problem 4: Buffer preparation mass

How many grams of NH₄Cl must be added to 2.40 L of 0.800 M NH₃ to make a buffer of pH 8.90? (Kb for NH₃ = 1.77×10⁻⁵)

Problem 5: Ratio for pH one unit below pKa

For a weak acid, what is the [A⁻]/[HA] ratio when pH is one unit below its pKa?

Solutions

Problem 1 Solution

1. Write the Henderson–Hasselbalch equation:
   pH = pKa + log([base]/[acid])
2. Substitute values:
   pH = 4.76 + log(0.100 M / 0.400 M)
3. Compute the ratio:
   0.100/0.400 = 0.250
4. Take the logarithm:
   log 0.250 = –0.602
5. Calculate pH:
   pH = 4.76 + (–0.602) = 4.158

Problem 2 Solution

1. Henderson–Hasselbalch can be rearranged to relate acid and base:
   pH = pKa + log([A⁻]/[HA])
2. Substitute pH and pKa:
   7.38 = 7.07 + log([A⁻]/[HA])
3. Solve for log ratio:
   log([A⁻]/[HA]) = 7.38 − 7.07 = 0.31
4. Convert from log:
   [A⁻]/[HA] = 10^0.31 = 2.04
5. Fraction undissociated (HA) = 1 / (1 + [A⁻]/[HA]) = 1 / (1 + 2.04) = 0.329
6. Percent undissociated = 32.9%

Problem 3 Solution

1. Initial moles in 0.500 L:
   total M = 0.800 M → 0.800 mol/L × 0.500 L = 0.400 mol (sum of HA + A⁻)
2. At pH = pKa, [HA] = [A⁻] = 0.200 mol each
3. Adding 0.100 mol HCl converts 0.100 mol A⁻ → HA:
   new A⁻ = 0.200 − 0.100 = 0.100 mol; new HA = 0.200 + 0.100 = 0.300 mol
4. Apply Henderson–Hasselbalch:
   pH = 4.752 + log(0.100/0.300) = 4.752 + log(0.333)
5. log(0.333) = –0.477
6. New pH = 4.752 – 0.477 = 4.275

Problem 4 Solution

1. For NH₃/NH₄⁺ buffer, use Henderson–Hasselbalch in base form:
   pOH = pKb + log([conj acid]/[base]) then pH = 14 – pOH
2. pKb = –log(1.77×10⁻⁵) = 4.752
3. Desired pH = 8.90 → pOH = 14.00 – 8.90 = 5.10
4. Substitute into pOH expression:
   5.10 = 4.752 + log([NH₄⁺]/[NH₃])
5. Solve for ratio:
   log([NH₄⁺]/[NH₃]) = 5.10 – 4.752 = 0.348 → [NH₄⁺]/[NH₃] = 10^0.348 = 2.23
6. Moles NH₃ initial = 0.800 M × 2.40 L = 1.92 mol
7. Let x = moles NH₄Cl added = moles NH₄⁺; then x/1.92 = 2.23 → x = 1.92×2.23 = 4.285 mol
8. Mass NH₄Cl = 4.285 mol × 53.49 g/mol = 229.3 g

Problem 5 Solution

1. Set pH = pKa – 1 → substitute into Henderson–Hasselbalch:
   pKa – 1 = pKa + log([A⁻]/[HA])
2. Rearranged:
   –1 = log([A⁻]/[HA]) → [A⁻]/[HA] = 10^(–1) = 0.1

Henderson-Hasselbalch Calculator